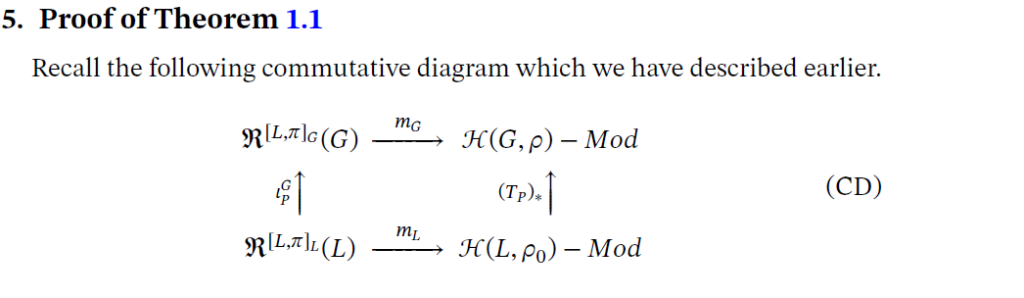The Department of Mathematics, SRM University-AP, is pleased to announce that Assistant Professor Dr Subha Sandeep Repaka has published a significant research paper titled “On Reducibility of Induced Representations of Odd Unitary Groups: The Depth Zero Case.” This accomplishment reflects Dr Repaka’s expertise and dedication to advancing mathematical research, further enriching the academic contributions of the department and the university.
Abstract:
We study a problem concerning parabolic induction in certain $p$-adic unitary groups. More precisely, for $E/F$ a quadratic extension of $p$-adic fields the associated unitary group $G=\mathrm{U}(n,n+1)$ contains a parabolic subgroup $P$ with Levi component $L$ isomorphic to $\mathrm{GL}_n(E) \times \mathrm{U}_1(E)$. Let $\pi$ be an irreducible supercuspidal representation of $L$ of depth zero. We use Hecke algebra methods to determine when the parabolically induced representation $\iota_P^G \pi$ is reducible.
Future Research Plans:
We would like to solve the same problem which I had solved in this paper for the groups U(n,n) and U(n,n+1) over p-adic fields using L-Functions which is a very novel approach.
The link to the article:
http://nyjm.albany.edu/j/2024/30-50.html